Q. 1 – Q. 45 carry one mark each, for which only one option is correct. Any wrong answer will be lead to deduction of 1/3 mark.
1. The equation of state of a gas is given by (P+aV3)(V−b2)=cT, where P, V, T are pressure, volume and temperature respectively, and a, b, c are constant. The dimensions of a and b are respectively
(A) ML8T−2 and L3/2 (B) ML5T−2 and L3 (C) ML5T−2 and L6 (D) ML6T−2 and L3/2
2. A capacitor of capacitance C0 is charged to a potential V0 and is connected with another capacitor of capacitance C as shown. After closing the switch S, the common potential across the two capacitors becomes V. The capacitance C is given by

(A) C0(V0−V)V0 (B) C0(V−V0)V0 (C) C0(V+V0)V (D) C0(V0−V)V
3. The r.m.s. speed of the molecules of a gas at 100°C is v. The temperature at which the r.m.s. speed will be √3v is
(A) 546°C (B) 646°C (C) 746°C (D) 846°C
4. As shown in the figure below, a charge +2C is situated at the origin O and another charge +5C is on the x-axis at the point A. The later charge from the point A is then brought to a point B on the y-axis. The work done is
(given 14πϵ0=9×109 m/F)
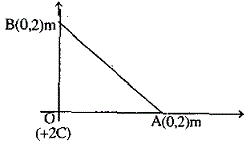
(A) 45×109 J (B) 90×109 J (C) Zero (D) −45×109 J
5. A frictionless piston-cylinder based enclosure contains some amount of gas at a pressure of 400 kPa. Then heat is transferred to the gas at constant pressure in a quasi-static process. The piston moves up slowly through a height of 10 cm. If the piston has a cross-sectional area of 0.3 m², the work done by the gas in this process is
(A) 6 kJ (B) 12 kJ (C) 7.5 kJ (D) 24 kJ
6. An electric cell of e.m.f. E is connected across a copper wire of diameter d and length l. The drift velocity of electrons in the wire is vd. If the length of the wire is changed to 2l, the new drift velocity of electrons in the copper wire will be
(A) vd (B) 2vd (C) vd/2 (D) vd/4
7. A NOR gate and a NAND gate are connected as shown in the figure. Two different sets of inputs are given to this set up. In the first case, the inputs to the gates are A=0, B=0, C=0. In the second case, the inputs are A=1, B=0, C=1. The output D in the first case and second case respectively are
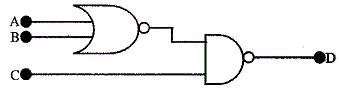
(A) 0 and 0 (B) 0 and 1 (C) 1 and 0 (D) 1 and 1
8. A bar magnet has a magnetic moment of 200 A.m². The magnet is suspended in a magnetic field of 0.30 NA-1m-1. The torque required to rotate the magnet from its equilibrium position through an angle of 30°, will be
(A) 30 N m (B) 30√3 N m (C) 60 N m (D) 60√3 N m
9. Two soap bubbles of radii r and 2r are connected by a capillary tube-valve arrangement as shown in the diagram. The valve is now opened. Then which one of the following will result:
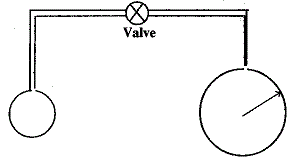
(A) the radii of the bubbles will remain unchanged
(B) the bubbles will have equal radii
(C) the radius of the smaller bubble will increase and that of the bigger bubble will decrease
(D) the radius of the smaller bubble will decrease and that of the bigger bubble will increase
10. An ideal mono-atomic gas of given mass is heated at constant pressure. In this process, the fraction of supplied heat energy used for the increase of the internal energy of the gas is
(A) 3/8 (B) 3/5 (C) 3/4 (D) 2/5
11. The velocity of a car travelling on a straight road is 36 kmh-1 at an instant of time. Now travelling with uniform acceleration for 10 s, the velocity becomes exactly double. If the wheel radius of the car is 25 cm, then which of the following numbers is the closest to the number of revolutions that the wheel makes during this 10 s ?
(A) 84 (B) 95 (C) 126 (D) 135
12. Two glass prisms P1 and P2 are to be combined together to produce dispersion without deviation. The angles of the prisms P1 and P2 are selected as 4° and 3° respectively. If the refractive index of prism P1 is 1.54, then that of P2 will be
(A) 1.48 (B) 1.58 (C) 1.62 (D) 1.72
13. The ionization energy of the hydrogen atom is 13.6 eV. The potential energy of the electron is n = 2 state of hydrogen atom is
(A) +3.4 eV (B) -3.4 eV (C) +6.8 eV (D) -6.8 eV
14. Water is flowing in streamline motion through a horizontal tube. The pressure at a point in the tube is p where the velocity of flow is v. At another point, where the pressure is p/2, the velocity of flow is [density of water = ρ]
(A) √v2+pρ (B) √v2−pρ (C) √v2+2pρ (D) √v2−2pρ
15. In the electrical circuit shown in figure, the current through the 4Ω resistor is
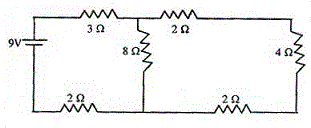
(A) 1 A (B) 0.5 A (C) 0.25 A (D) 0.1 A
16. A wire of initial length L and radius r is stretched by a length l. Another wire of same material but with initial length 2L and radius 2r is stretched by a length 2l. The ratio of the stored elastic energy per unit volume in the first and second wire is,
(A) 1 : 4 (B) 1 : 2 (C) 2 : 1 (D) 1 : 1
17. A current of 1 A is flowing along positive x-axis through a straight wire of length 0.5 m placed in a region of a magnetic field given by →B=(2ˆi+4ˆj)T. The magnitude and the direction of the force experienced by the wire respectively are
(A) √18 N, along positive z-axis
(B) √20 N, along positive x-axis
(C) 2N, along positive z-axis
(D) 4N, along positive y-axis
18. Two spheres of the same material, but of radii R and 3R are allowed to fall vertically downwards through a liquid of density σ. The ratio of their terminal velocities is
(A) 1 : 3 (B) 1 : 6 (C) 1 : 9 (D) 1 : 1
19. S1 and S2 are the two coherent source of light located in the xy-plane at points (0,0) and (0,3λ) respectively. Here λ is the wavelength of light. At which one of the following points (given as coordinates), the intensity of interference will be maximum ?
(A) (3λ,0) (B) (4λ,0) (C) (5λ/4,0) (D) (2λ/3,0)
20. An alpha particle (4He) has a mass of 4.00300 amu. A proton has mass of 1.00783 amu and a neutron has mass of 1.00867 amu respectively. The binding energy of alpha particle estimated from these data is closest to
(A) 27.9 MeV (B) 22.3 MeV (C) 35.0 MeV (D) 20.4 MeV
21. Four small objects each of mass m are fixed at the corners of a rectangular wire-frame of negligible mass and of sides a and b (a > b). If the wire frame is now rotated about an axis passing along the side of length b, then the moment of inertia of the system for this axis of rotation is
(A) 2ma² (B) 4ma² (C) 2m(a² + b²) (D) 2m(a² - b²)
22. The equivalent resistance between the points a and b of the electrical network shown in the figure is
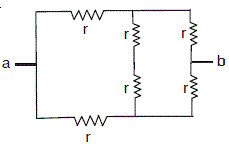
(A) 6r (B) 4r (C) 2r (D) r
23. The de Broglie wavelength of an electron (mass = 1 x 10-30 kg, charge = 1.6 x 10-19 C) with a kinetic energy of 200 eV is (Planck's constant = 6.6 x 10-34 J s)
(A) 9.60 x 10-11 m (B) 8.25 x 10-11 m (C) 6.25 x 10-11 m (D) 5.00 x 10-11 m
24. An object placed at a distance of 16 cm from a convex lens produces an image of magnification m (m > 1). If the object is moved towards the lens by 8 cm then again an image of magnification m is obtained. The numerical value of the focal length of the lens is
(A) 12 cm (B) 14 cm (C) 18 cm (D) 20 cm
25. The number of atoms of a radioactive substance of half-life T is N0 at t = 0. The time necessary to decay from N0/2 atoms to N0/10 atoms will be
(A) 52T (B) Tln5 (C) Tln(52) (D) Tln5ln2
26. A travelling acoustic wave of frequency 500 Hz is moving along the positive x-direction with a velocity of 300 ms-1. The phase difference between two points x1 and x2 is 60°. Then the minimum separation between the two points is
(A) 1 mm (B) 1 cm (C) 10 cm (D) 1 m
27. A mass M at rest is broken into two pieces having masses m and (M-m). The two masses are then separated by a distance r. The gravitational force between them will be the maximum when the ratio of the masses [m:(M-m)] of the two parts is
(A) 1 : 1 (B) 1 : 2 (C) 1 : 3 (D) 1 : 4
28. A shell of mass 5M, acted upon by no external force and initially at rest, bursts into three fragments of masses M, 2M and 2M respectively. The first two fragments move in opposite directions with velocities of magnitudes 2V and V respectively. The third fragment will
(A) move with a velocity V in a direction perpendicular to other two
(B) move with a velocity 2V in the direction of velocity of the first fragment
(C) be at rest
(D) move with a velocity V in the direction of velocity of the second fragment
29. A bullet of mass m travelling with a speed v hits a block of mass M initially at rest and gets embedded in it. The combined system is free to move and there is no other force acting on the system. The heat generated in the process will be
(A) Zero (B) mv22 (C) Mmv22(M−m) (D) mMv22(M+m)
30. A particle moves along X-axis and its displacement at any time is given by x(t) = 2t³ - 3t² + 4t in SI units. The velocity of the particle when its acceleration is zero, is
(A) 2.5 ms-1 (B) 3.5 ms-1 (C) 4.5 ms-1 (D) 8.5 ms-1
31. A planet moves around the sun in a elliptical orbit with the sun at one of its foci. The physical quantity associated with the motion of the planet that remains constant with time is
(A) velocity (B) centripetal force (C) linear momentum (D) angular momentum
32. The fundamental frequency of a closed pipe is equal to the frequency of the second harmonic of an open pipe. The ratio of their lengths is
(A) 1 : 2 (B) 1 : 4 (C) 1 : 8 (D) 1 : 16
33. A particle of mass M and charge q is released from rest in a region of uniform electric field of magnitude E. After a time t, the distance travelled by the charge is S and the kinetic energy attained by the particle is T, then the ratio T/S
(A) remains constant with time t
(B) varies linearly with the mass M of the particle
(C) is independent of the charge q
(D) is independent of the magnitude of the electric field E
34 . An alternating current in a circuit is given by I=20sin(100πt+0.05π) A. The r.m.s value and the frequency of current respectively are,
(A) 10 A & 100 Hz (B) 10 A & 50 Hz (C) 10√2 A & 50 Hz (D) 10√2 A & 100 Hz
35. The specific heat c of a solid at low temperature shows temperature dependence according to the relation c=DT³ where D is a constant and T is the temperature in kelvin. A piece of this solid of mass m kg is taken and its temperature is raised from 20 K to 30 K. The amount of heat required in the process in energy units is
(A) 5 x 104 Dm (B) (33/4) x 104 Dm (C) (65/4) x 104 Dm (D) (5/4) x 104 Dm
36. Four identical plates each of area a are separated by a distance d. The connection is shown below. What is the capacitance between P and Q ?

(A) 2aϵ0/d (B) aϵ0/(2d) (C) aϵ0/d (D) 4aϵ0/d
37. The least distance of vision of a longsighted person is 60 cm. By using a spectacle lens this distance is reduced to 12 cm. The power of the lens is
(A) +5.0 D (B) +(20/3) D (C) -(10/3) (D) D +2.0 D
38. A particle is acted upon by a constant power. Then, which of the following physical quantity remains constant ?
(A) speed
(B) rate of change of acceleration
(C) kinetic energy
(D) rate of change kinetic energy
39. A particle of mass M and charge q, initially at rest is accelerated by a uniform electric field E through a distance D and is then allowed to approach a fixed static charge Q of the same sign. The distance of the closest approach of the charge q will then be
(A) qQ4πϵ0D (B) Q4πϵ0ED (C) qQ2πϵ0D2 (D) Q4πϵ0E
40. In an n-p-n transistor
(A) the emitter has higher degree of doping compared to that of the collector
(B) the collector has higher degree of doping compared to that of the emitter
(C) both the emitter and collector have same degree of doping
(D) the base region is most heavily doped
41. At two different places the angles of dip are respectively 30° and 45°. At these two places the ratio of horizontal component of earth's magnetic field is
(A) √3 : √2 (B) 1 : √2 (C) 1 : 2 (D) 1 : √3
42. Two vectors are given by →A=ˆi+2ˆj+2ˆk and →B=3ˆi+6ˆj+2ˆk. Another vector →C has the same magnitude as →B but has the same direction as →A. Then which of the following vectors represents →C ?
(A) 73(ˆi+2ˆj+2ˆk) (B) 37(ˆi−2ˆj+2ˆk) (C) 79(ˆi−2ˆj+2ˆk) (D) 97(ˆi+2ˆj+2ˆk)
Ans : (A)
43. An equilateral triangle is made by uniform wires AB, BC, CA. A current I enters at A and leaves from the mid point of BC. If the lengths of each side of the triangle is L, the magnetic field B at centroid O of the triangle is
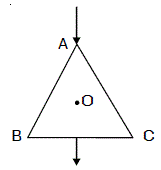
(A) μ04π(4IL) (B) μ02π(4IL) (C) μ04π(2IL) (D) Zero
44. A car moving at a velocity of 17 ms-1 towards an approaching bus that blows a horn at a frequency of 640 Hz on a straight track. The frequency of this horn appears to be 680 Hz to the car driver. If the velocity of sound in air is 340 ms-1, then the velocity of the approaching bus is
(A) 2 ms-1 (B) 4 ms-1 (C) 8 ms-1 (D) 10 ms-1
45. A particle is moving with a uniform speed v in a circular path of radius r with the centre at O. When the particle moves from a point P to Q on the circle such that ∠POQ=θ, then the magnitude of the change in velocity is
(A) 2vsin(2θ) (B) Zero (C) 2vsin(θ2) (D) 2vcos(θ2)
Q. 46 – Q. 55 carry two marks each, for which only one option is correct. Any wrong answer will lead to deduction of 2/3 mark
46. Two simple harmonic motions are given by
x1=asinωt+acosωt and
x2=asinωt+a√3cosωt
The ratio of the amplitudes of first and second motion and the phase difference between them are respectively
(A) √32 and π12 (B) √32 and π12 (C) 2√3 and π12 (D) √32 and π6
47. A small mass m attached to one end of a spring with a negligible mass and an unstretched length L, executes vertical oscillations with angular frequency ω0, when the mass is rotated with an angular speed ω by holding the other end of the spring at a fixed point, the mass moves uniformly in a circular path in a horizontal plane. Then the increase in length of the spring during this rotation is
(A) ω2Lω20−ω2 (B) ω20Lω2−ω20 (C) ω2Lω20 (D) ω20Lω2
48. A cylindrical block floats vertically in a liquid of density ρ1 kept in a container such that the fraction of volume of the cylinder inside the liquid is x1. Then some amount of another immiscible liquid of density ρ2 (ρ2<ρ1) is added to the liquid in the container so that the cylinder now floats just fully immersed in the liquids with x2 fraction of volume of the cylinder insider the liquid density ρ1. The ratio ρ1/ρ2 will be
(A) 1−x2x1−x2 (B) 1−x1x1+x2 (C) x1−x2x1+x2 (D) x2x1−1
49. A sphere of radius R has a volume density of charge ρ=kr, where r is the distance from the centre of the sphere and k is constant. The magnitude of the electric field which exists at the surface of the sphere is given by (ϵ0 = permittivity of the free space)
(A) 4πkR43ϵ0 (B) kR3ϵ0 (C) 4πkRϵ0 (D) kR24ϵ0
50. A particle of mass M and charge q is at rest at the midpoint between two other fixed similar charges each of magnitude Q placed a distance 2d apart. The system is collinear as shown in the figure. The particle is now displaced by a small amount x (x << d) along the line joining the two charges and is left to itself. It will now oscillate about the mean position with a time period (ϵ0 = permittivity of the free space)
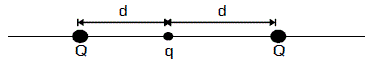
(A) 2√π3Mϵ0dQq (B) 2√π2Mϵ0d3Qq (C) 2√π3Mϵ0d3Qq (D) 2√π3Mϵ0Qqd3
51. A body is projected from the ground with a velocity →v=(3ˆi+10ˆj) ms-1. The maximum height attained and the range of the body respectively are (given g = 10 ms-2)
(A) 5 m and 6 m (B) 3 m and 10 m (C) 6 m and 5 m (D) 3 m and 5 m
52. The stopping potential for photoelectrons from a metal surface is V1 when monochromatic light of frequency ν1 is incident on it. The stopping potential becomes V2 when monochromatic light of another frequency is incident on the same metal surface. Of h be the Planck's constant and e be the charge of an electron, then the frequency of light in the second case is
(A) ν1−eh(V2+V1) (B) ν1+eh(V2+V1) (C) ν1−eh(V2−V1) (D) ν1+eh(V2−V1)
53. A cell of e.m.f. E is connected to a resistance R1 for time t and the amount of heat generated in it is H. If the resistance R1 is replaced by another resistance R2 and is connected to the cell for the same time t, the amount of heat generated in R2 is 4H. Then the internal resistance of the cell is
(A) 2R1+R22
(B) √R1R22√R2−√R1√R2−2√R1
(C) √R1R2√R2−2√R12√R2−√R1
(D) √R1R2√R2−√R1√R2+√R1
54. 3 moles of a mono-atomic gas (γ = 5/3) is mixed with 1 mole of a diatomic gas (γ = 7/3). The value of γ for the mixture will be
(A) 9/11 (B) 11/7 (C) 12/7 (D) 15/7
55. The magnetic field B=2t2+4t2 (where t = time) is applied perpendicular to the plane of a circular wire of radius r and resistance R. If all the units are in SI the electric charge that flows through the circular wire during t = 0 s to t = 2 s is
(A) 6πr2R (B) 20πr2R (C) 32πr2R (D) 48πr2R
Q. 56 – Q. 60 carry two marks each, for which one or more than one options may be correct. Marking of correct options will lead to a maximum mark of twoon pro rata basis. There willbe no negative marking for these questions. However, any marking of wrong option will lead to award of zero mark against the respective question – irrespective of the number of corredt options marked.
56. If E and B are the magnitudes of electric and magnetic fields respectively in some region of space, then the possibilities for which a changed particle may move in that space with a uniform velocity of magnitude v are
(A) E = vB (B) E ≠ 0, B = 0 (C) E = 0, B ≠ 0 (D) E ≠ 0, B ≠ 0
57. An electron of charge e and mass m is moving in circular path of radius r with a uniform angular speed ω. Then which of the following statements are correct ?
(A) The equivalent current flowing in the circular path is proportional to r²
(B) The magnetic moment due to circular current loop is independent of m
(C) The magnetic moment due to circular current loop is equal to 2e/m time the angular momentum of the electron
(D) The angular momentum of the particle is proportional to the areal velocity of electron
58. A biconvex lens of focal length f and radii of curvature of both the surfaces R is made of a material of refractive index n1. This lens is placed in a liquid of refractive index n2. Now this lens will behave like
(A) either as a convex or as a concave lens depending solely on R
(B) a convex lens depending on n1 and n2
(C) a concave lens depending on n1 and n2
(D) a convex lens of same focal length irrespective of R, n1 and n2
59. A block of mass m (m = 0.1 kg) is hanging over a frictionless light fixed pulley by an inextensible string of negligible mass. The other end of the string is pulled by a constant force F in the vertically downward direction. The linear momentum of the block increase by 2 kg ms-1 in 1 s after the block starts from rest. Then, (given g = 10 ms-2)
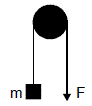
(A) The tension in the string is F
(B) The tension in the string is 3N
(C) The work done by the tension on the block is 20 J during this 1s
(D) The work done against the force of gravity is 10 J
60. A bar of length l carrying a small mass m at one of its ends rotates with a uniform angular speed ω in a vertical plane about the mid-point of the bar. During the rotation, at some instant of time when the bar is horizontal, the mass is detached from the bar but the bar continues to rotate with same ω. The mass moves vertically up, comes back and reaches the bar at the same point. At that place, the acceleration due to gravity is g.
(A) This is possible of the quantity ω2l2πg is an integer
(B) The total time of flight of the mass is proportional to ω²
(C) The total distance travelled by the mass in air is proportional to ω²
(D) The total distance travelled by the mass in air and its total time of flight are both independent on its mass
***










